Know the Impact of Mathematical Symmetry in Daily Life – Importance of Patterns
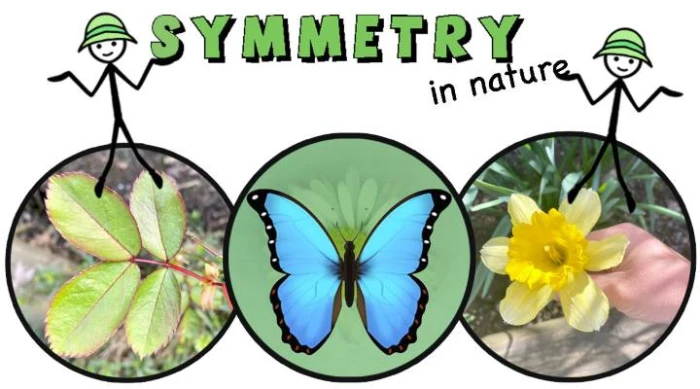
The most apparent examples of symmetry can be found in the natural world. From a snowflake’s intricate design to a sunflower’s radial symmetry, nature thrives on balance.
One of the most beautiful things about universe is the symmetry and Math is like the language of the universe. Symmetry means something can be divided into two or more equal parts. We can see symmetry everywhere, in nature, arts, buildings, and even in our daily lives. Studying patterns and symmetry helps us to understand the world better. It’s also important for many things such as design and technology.
Let’s talk about the fact that in our everyday lives math can be seen in small and big things. And in this guide, we’ll see that how math helps us recognize patterns and how these patterns are important.
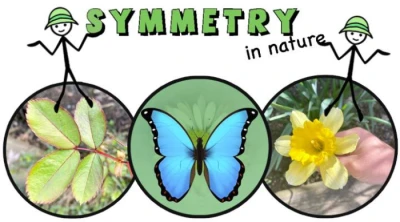
Symmetry in Nature
The most apparent examples of symmetry can be found in the natural world. From a snowflake’s intricate design to a sunflower’s radial symmetry, nature thrives on balance and repetition. Symmetry is often an indicator of health and functionality in biological organisms. For example, animals with symmetrical faces and bodies are generally perceived to be healthier and more genetically fit, a concept that has even been linked to evolutionary biology and mate selection.
Plants also display symmetry especially in their leaves, flowers, and seeds. The spiral pattern seen in the arrangement of leaves or in the scales of a pine cone is a type of symmetry known as the Fibonacci sequence, named after the famous Italian mathematician. This pattern optimizes space and sunlight exposure, demonstrating how natural systems utilize mathematical principles for survival.
The human body is another example of bilateral symmetry, meaning our left and right sides mirror each other. This symmetry affects our appearance and function. Balance, posture, and even certain psychological perceptions are closely related to the symmetry of our bodies. Recognizing these symmetrical patterns allows scientists and doctors to understand health condition and guide treatments better.
Symmetry in Art and Architecture
Human-made structures, too, are built around the concept of symmetry. Historically, architects have used symmetrical designs to create visually appealing and structurally sound buildings. Consider the Parthenon in Greece or the Taj Mahal in India, both are striking examples of symmetry in architecture which is aesthetically pleasing and functional. Symmetry ensures balance which is crucial in engineering and construction.
In art, symmetry often conveys harmony and order. Many cultures have used symmetrical patterns in their artwork to reflect their ideas of beauty and spirituality. Islamic art, for instance, is known for its use of intricate geometric patterns based on mathematical symmetry principles. These patterns symbolize the infinite nature of God as the repeating designs suggest an unending pattern.
Symmetry in music, too, plays an essential role. For instance, in classical compositions, symmetrical notes’ arrangements create balance and predictability, making the music more pleasant for the human ear. This concept of symmetry also extends to literature where balanced structures in poetry and prose create rhythm and flow enhancing the overall reading experience.
The Importance of Recognizing Patterns
Beyond visual and aesthetic application, patterns, and symmetry are crucial for problem-solving and innovation. Recognizing patterns helps us make sense of the world around us. It allows us to predict outcomes, streamline processes, and innovate in various fields including science, technology, and economics.
In mathematics, symmetry is a tool for solving complex problems. Whether it’s balancing equations or optimizing some algorithms, mathematicians often rely on symmetry to simplify their work. For instance, in physics, the laws of motion and conservation are based on symmetrical principles. The symmetry of physical laws ensures that the same rules apply, whether you’re on Earth or in space, thus enabling scientists to make accurate predictions about the behavior of matter.
In computer science, algorithms often depend on patterns and symmetrical arrangements to function efficiently. Sorting algorithms which are used in everything from search engines to financial trading, utilize symmetry to organize data quickly and accurately. AI systems are designed to identify patterns in data, enabling them to make decisions, solve problems, and even predict future trends. By understanding the importance of symmetry and patterns, AI developers can create more efficient and accurate algorithms that enhance technology’s role in our lives.
Symmetry in Daily Life
The influence of symmetry in our daily lives is often subtle but profound, for example, we can see symmetry in fashion. The symmetrical design of clothing, whether it’s the balance of a collar, the cut of a jacket, or the matching sides of a dress, enhances visual appeal and provides comfort to the wearer. In interior design, symmetry is often used to create a sense of order and tranquility. A well-balanced room feels more harmonious and inviting.
Symmetry also affects how we perceive time. Patterns in timekeeping such as the symmetry of clocks and calendars, help us organize our schedules and plan our activities. The regularity of the day-night cycle, seasons, and yearly events are examples of temporal symmetry that structure our lives. In our daily routines, we constantly rely on patterns. Whether it’s the route we take for work, how we recognize our tasks, or even how we communicate, patterns help us navigate the complexities of modern life. Recognizing symmetry and patterns allows us to operate more efficiently, make better decisions, and solve problems more effectively.
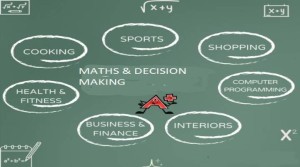
Let’s explore the different aspects of mathematical symmetry and how it shapes our daily lives, focusing on shape symmetry, rotational symmetry, symmetric algebra, symmetry and group theory, and geometric symmetry.
Shape Symmetry
Shape symmetry is the most commonly observed form of symmetry which refers to a scenario where one half of an object or shape is a mirror image of the other half. This type of symmetry is called reflective symmetry or mirror symmetry. Whether it’s a butterfly’s wings, a human face, or a leaf from a plant, shape symmetry is an inherent part of the natural world. In our daily life, we see shape symmetry everywhere.
Consider objects like furniture, clothing, or road signs; symmetry provides balance and stability. When something is symmetrical, it is easier to design and construct because one part replicates another, simplifying complex processes. Shape symmetry is also essential in various artistic disciplines including architecture and design. Many people find these structures attractive because of their pleasing and balanced design.
Use our scientific calculator and statistics calculator to have more grip on your mathematical calculations.
Rotational Symmetry
Rotational symmetry refers to an object that looks the same after being rotated by a certain angle. A common example in daily life is the wheel of a car. When the wheel spins, it maintains its symmetry at different angles. This property is important in the design of various objects like machinery and tools but also appears in nature such as flowers that look the same when rotated at different angles. In mathematics, rotational symmetry helps in understanding geometric shapes and their properties. This principle is applied in various fields including physics where the laws of nature remain invariant under rotation and in engineering where gears, turbines, and other rotating machinery depend on symmetry for efficient function. Rotational symmetry is also evident in cultural artifacts and art. Mandalas, circular designs found in various spiritual traditions exhibit intricate rotational symmetry offering aesthetic beauty and symbolic significance.
Symmetric Algebra
Symmetric algebra extends the concept of symmetry into the world of algebra. Symmetry in algebra relates to operations and functions that remain unchanged under certain transformations. For example, in solving equations, symmetric functions simplify complex problems. This symmetry helps mathematicians and scientists solve polynomial equations by making them easier to manage through systematic approaches. Symmetric algebra is also crucial in computer science, particularly in algorithm design. This has vast implications for coding and data encryption where maintaining structural symmetry is essential for security.
Symmetry and Group Theory
Group theory is a branch of mathematics that studies the algebraic structures known as groups which are collections of elements that combine according to specific rules. Symmetry is a fundamental concept in group theory because many of the problems it addresses involve symmetry in some form. For example, group theory helps mathematicians describe the symmetries of geometric objects and understand how these symmetries interact. Group theory and symmetry are essential in various fields like cryptography where data security relies on complex mathematical structures.
Group theory is also used to study the structure of molecules in chemistry. How atoms are arranged in a molecule can affect its chemical properties. Group theory is also crucial in physics especially in understanding the symmetries of physical laws. The theory helps explain fundamental forces and particles in the universe through symmetry. This connection between symmetry and the physical world allows scientists to develop models explaining matter and energy behavior.
Geometric Symmetry
Geometric symmetry refers to the symmetry of geometric shapes and figures which is often analyzed through transformations like translation, rotation, reflection, and scaling. Geometric symmetry is fundamental in fields such as architecture, engineering, and arts. For instance, in construction, symmetrical designs provide both aesthetic appeal and practical stability. Symmetrical buildings, bridges, and other structures distribute weight evenly, preventing imbalances that could lead to collapse. Geometric symmetry isn’t limited to artificial structures.
Nature offers countless examples of geometric symmetry such as honeycombs’ hexagonal symmetry or shells’ spiral symmetry. These patterns are beautiful and efficient allowing nature to use resources in the most effective way possible. In mathematics, geometric symmetry helps solve problems related to shapes and spatial understanding. The study of symmetry in geometry allows mathematicians to classify shapes, predict their behavior under transformations and solve complex issues related to tiling, tessellations, and space-filling patterns.
Symmetry is more than just a mathematical concept, it is an intrinsic part of our daily experience. From the natural world to human-made designs, symmetry gives structure, balance, and efficiency to what we see and use daily. In nature, symmetry ensures survival by allowing organisms to develop evenly and maintain balance. In human creations, symmetry enhances aesthetics, functionality, and stability whether in arts, architecture, or technology.
Mathematically, symmetry helps simplify problems, making it easier to solve equations, understand physical phenomena, and design systems that work efficiently. Whether through group theory, algebra, or geometry, the study of symmetry continues to influence various fields, from science to engineering to the arts. In essence, symmetry connects the abstract world of mathematics with the tangible reality of our surroundings, making it an indispensable part of both human life and nature’s designs.
One of the most beautiful things about universe is the symmetry and Math is like the language of the universe. Symmetry means something can be divided into two or more equal parts. We can see symmetry everywhere, in nature, arts, buildings, and even in our daily lives. Studying patterns and symmetry helps us to understand the world better. It’s also important for many things such as design and technology.
Let’s talk about the fact that in our everyday lives math can be seen in small and big things. And in this guide, we’ll see that how math helps us recognize patterns and how these patterns are important.
Symmetry in Nature
The most apparent examples of symmetry can be found in the natural world. From a snowflake’s intricate design to a sunflower’s radial symmetry, nature thrives on balance and repetition. Symmetry is often an indicator of health and functionality in biological organisms. For example, animals with symmetrical faces and bodies are generally perceived to be healthier and more genetically fit, a concept that has even been linked to evolutionary biology and mate selection.
Plants also display symmetry especially in their leaves, flowers, and seeds. The spiral pattern seen in the arrangement of leaves or in the scales of a pine cone is a type of symmetry known as the Fibonacci sequence, named after the famous Italian mathematician. This pattern optimizes space and sunlight exposure, demonstrating how natural systems utilize mathematical principles for survival.
The human body is another example of bilateral symmetry, meaning our left and right sides mirror each other. This symmetry affects our appearance and function. Balance, posture, and even certain psychological perceptions are closely related to the symmetry of our bodies. Recognizing these symmetrical patterns allows scientists and doctors to understand health condition and guide treatments better.
Symmetry in Art and Architecture
Human-made structures, too, are built around the concept of symmetry. Historically, architects have used symmetrical designs to create visually appealing and structurally sound buildings. Consider the Parthenon in Greece or the Taj Mahal in India, both are striking examples of symmetry in architecture which is aesthetically pleasing and functional. Symmetry ensures balance which is crucial in engineering and construction.
In art, symmetry often conveys harmony and order. Many cultures have used symmetrical patterns in their artwork to reflect their ideas of beauty and spirituality. Islamic art, for instance, is known for its use of intricate geometric patterns based on mathematical symmetry principles. These patterns symbolize the infinite nature of God as the repeating designs suggest an unending pattern.
Symmetry in music, too, plays an essential role. For instance, in classical compositions, symmetrical notes’ arrangements create balance and predictability, making the music more pleasant for the human ear. This concept of symmetry also extends to literature where balanced structures in poetry and prose create rhythm and flow enhancing the overall reading experience.
The Importance of Recognizing Patterns
Beyond visual and aesthetic application, patterns, and symmetry are crucial for problem-solving and innovation. Recognizing patterns helps us make sense of the world around us. It allows us to predict outcomes, streamline processes, and innovate in various fields including science, technology, and economics.
In mathematics, symmetry is a tool for solving complex problems. Whether it’s balancing equations or optimizing some algorithms, mathematicians often rely on symmetry to simplify their work. For instance, in physics, the laws of motion and conservation are based on symmetrical principles. The symmetry of physical laws ensures that the same rules apply, whether you’re on Earth or in space, thus enabling scientists to make accurate predictions about the behavior of matter.
In computer science, algorithms often depend on patterns and symmetrical arrangements to function efficiently. Sorting algorithms which are used in everything from search engines to financial trading, utilize symmetry to organize data quickly and accurately. AI systems are designed to identify patterns in data, enabling them to make decisions, solve problems, and even predict future trends. By understanding the importance of symmetry and patterns, AI developers can create more efficient and accurate algorithms that enhance technology’s role in our lives.
Symmetry in Daily Life
The influence of symmetry in our daily lives is often subtle but profound, for example, we can see symmetry in fashion. The symmetrical design of clothing, whether it’s the balance of a collar, the cut of a jacket, or the matching sides of a dress, enhances visual appeal and provides comfort to the wearer. In interior design, symmetry is often used to create a sense of order and tranquility. A well-balanced room feels more harmonious and inviting.
Symmetry also affects how we perceive time. Patterns in timekeeping such as the symmetry of clocks and calendars, help us organize our schedules and plan our activities. The regularity of the day-night cycle, seasons, and yearly events are examples of temporal symmetry that structure our lives. In our daily routines, we constantly rely on patterns. Whether it’s the route we take for work, how we recognize our tasks, or even how we communicate, patterns help us navigate the complexities of modern life. Recognizing symmetry and patterns allows us to operate more efficiently, make better decisions, and solve problems more effectively.
Let’s explore the different aspects of mathematical symmetry and how it shapes our daily lives, focusing on shape symmetry, rotational symmetry, symmetric algebra, symmetry and group theory, and geometric symmetry.
Shape Symmetry
Shape symmetry is the most commonly observed form of symmetry which refers to a scenario where one half of an object or shape is a mirror image of the other half. This type of symmetry is called reflective symmetry or mirror symmetry. Whether it’s a butterfly’s wings, a human face, or a leaf from a plant, shape symmetry is an inherent part of the natural world. In our daily life, we see shape symmetry everywhere.
Consider objects like furniture, clothing, or road signs; symmetry provides balance and stability. When something is symmetrical, it is easier to design and construct because one part replicates another, simplifying complex processes. Shape symmetry is also essential in various artistic disciplines including architecture and design. Many people find these structures attractive because of their pleasing and balanced design.
Use our scientific calculator and statistics calculator to have more grip on your mathematical calculations.
Rotational Symmetry
Rotational symmetry refers to an object that looks the same after being rotated by a certain angle. A common example in daily life is the wheel of a car. When the wheel spins, it maintains its symmetry at different angles. This property is important in the design of various objects like machinery and tools but also appears in nature such as flowers that look the same when rotated at different angles. In mathematics, rotational symmetry helps in understanding geometric shapes and their properties. This principle is applied in various fields including physics where the laws of nature remain invariant under rotation and in engineering where gears, turbines, and other rotating machinery depend on symmetry for efficient function. Rotational symmetry is also evident in cultural artifacts and art. Mandalas, circular designs found in various spiritual traditions exhibit intricate rotational symmetry offering aesthetic beauty and symbolic significance.
Symmetric Algebra
Symmetric algebra extends the concept of symmetry into the world of algebra. Symmetry in algebra relates to operations and functions that remain unchanged under certain transformations. For example, in solving equations, symmetric functions simplify complex problems. This symmetry helps mathematicians and scientists solve polynomial equations by making them easier to manage through systematic approaches. Symmetric algebra is also crucial in computer science, particularly in algorithm design. This has vast implications for coding and data encryption where maintaining structural symmetry is essential for security.
Symmetry and Group Theory
Group theory is a branch of mathematics that studies the algebraic structures known as groups which are collections of elements that combine according to specific rules. Symmetry is a fundamental concept in group theory because many of the problems it addresses involve symmetry in some form. For example, group theory helps mathematicians describe the symmetries of geometric objects and understand how these symmetries interact. Group theory and symmetry are essential in various fields like cryptography where data security relies on complex mathematical structures.
Group theory is also used to study the structure of molecules in chemistry. How atoms are arranged in a molecule can affect its chemical properties. Group theory is also crucial in physics especially in understanding the symmetries of physical laws. The theory helps explain fundamental forces and particles in the universe through symmetry. This connection between symmetry and the physical world allows scientists to develop models explaining matter and energy behavior.
Geometric Symmetry
Geometric symmetry refers to the symmetry of geometric shapes and figures which is often analyzed through transformations like translation, rotation, reflection, and scaling. Geometric symmetry is fundamental in fields such as architecture, engineering, and arts. For instance, in construction, symmetrical designs provide both aesthetic appeal and practical stability. Symmetrical buildings, bridges, and other structures distribute weight evenly, preventing imbalances that could lead to collapse. Geometric symmetry isn’t limited to artificial structures.
Nature offers countless examples of geometric symmetry such as honeycombs’ hexagonal symmetry or shells’ spiral symmetry. These patterns are beautiful and efficient allowing nature to use resources in the most effective way possible. In mathematics, geometric symmetry helps solve problems related to shapes and spatial understanding. The study of symmetry in geometry allows mathematicians to classify shapes, predict their behavior under transformations and solve complex issues related to tiling, tessellations, and space-filling patterns.
Symmetry is more than just a mathematical concept, it is an intrinsic part of our daily experience. From the natural world to human-made designs, symmetry gives structure, balance, and efficiency to what we see and use daily. In nature, symmetry ensures survival by allowing organisms to develop evenly and maintain balance. In human creations, symmetry enhances aesthetics, functionality, and stability whether in arts, architecture, or technology.
Mathematically, symmetry helps simplify problems, making it easier to solve equations, understand physical phenomena, and design systems that work efficiently. Whether through group theory, algebra, or geometry, the study of symmetry continues to influence various fields, from science to engineering to the arts. In essence, symmetry connects the abstract world of mathematics with the tangible reality of our surroundings, making it an indispensable part of both human life and nature’s designs.
Conversation
Latest Blogs
© Blog CoolCalculator, Explore CoolCalculator, your destination for the latest insights, tips, and updates on the world of online calculators. Stay informed and make your calculations smarter with our blog. ,
Designed
by Saad Media Team , Team Lead M.Rizwan Akhtar